
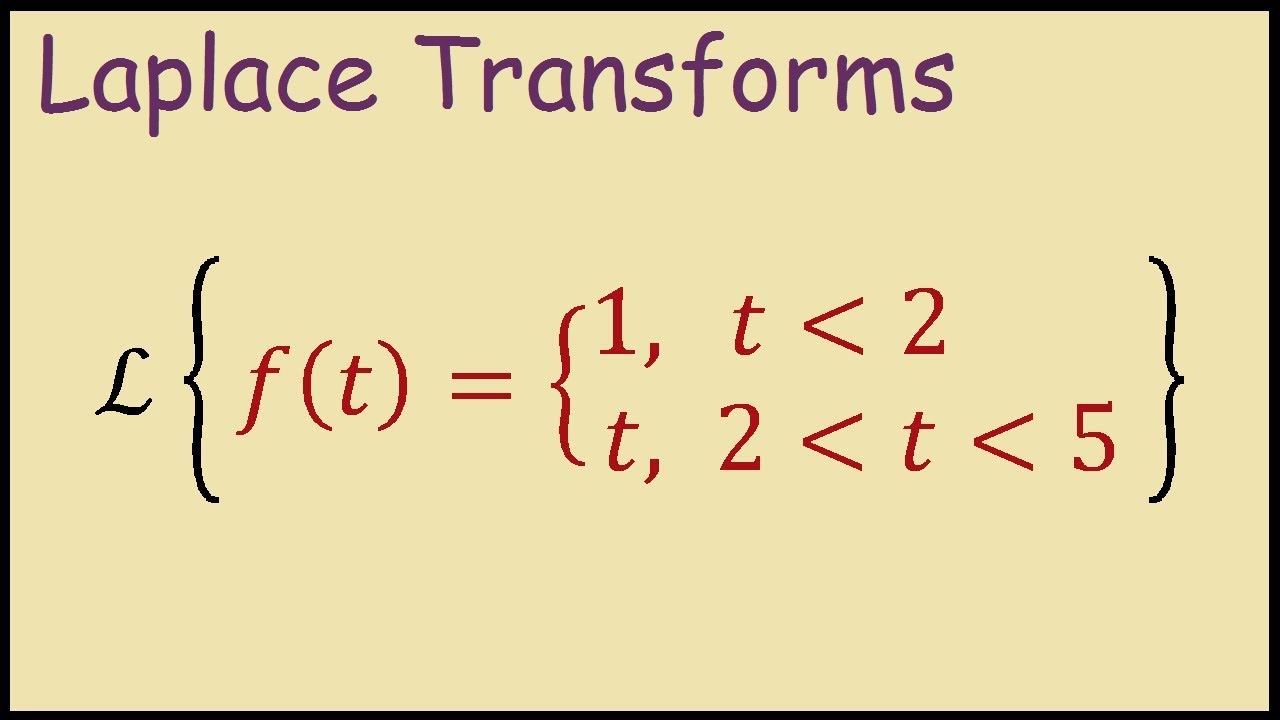

The notation of Laplace transform is an L-like symbol used to transform one function into another. It is an improper integral from zero to infinity of e to the minus st times f of t with respect to t. Hello, I tried to compute Laplace transform(sin(3t-2)e(-2t)) using WolframAlpha and I see no step-by-step solution. Laplace transform is a method to convert the given function into some other function of s. A piecewise Laplace transform calculator is a calculator used to find out the s-domain complex solution for a piecewise time domain signal which is not.
#LAPLACE TRANSFORM CALCULATOR WITH STEPS FREE#

Calculate the inverse Laplace transform, which will be your final solution to the original differential equation.The Laplace transform calculator is used to convert the real variable function to a complex-valued function. Use the formula learned in this section to turn all Laplace equations into the form L equated to something.ĥ.Take the Laplace Transform of the differential equation.The description and step by step process is given in the next list: The trick for this process, and which makes it very simple to follow, is that you will only be using the Laplace transform to decompose the differential equations into their different terms and simplify them once the Laplace transform has done this, you will take the inverse transformation to go and retrieve y(t). Since we will be solving "differential" equations, this means we will be working with derivatives of y, and so let us work through the Laplace transform of the first derivative of y:Įquation 6: Laplace transform of the first derivative of y and Laplace transform of the second derivative of y.
#LAPLACE TRANSFORM CALCULATOR WITH STEPS HOW TO#
If you need a review on how to use this table do not forget to check the lesson on calculating Laplace transforms.īut the most important part of the process of solving differential equations with a Laplace transform comes from a derivation of equation 1 shown in the last section, this is, when we apply the Laplace transform formula to the derivative of y.įrom the definition of Laplace transform given in equation 1, you can see that the represented transformation is of a function of t, this is because we usually define y=f(t) or "the y function is in terms of t, and therefore is a function of t". Such table containing common Laplace transforms will allow us to once again solve difficult problems by comparison with the equations contained on the table. This process will be aided by the Laplace transform table shown below: In reality, we need both Laplace transforms and Inverse Laplace transforms in order to find the solution to an ordinary differential equation, the trick is to apply one first (which will allow us to change the differential equation to an expression containing only y's), simplify the equation as much as possible and then reverse it by taking the inverse transformation to solve to y. Now is time to see how these transformations are helpful to us while solving differential equations. So we have already had an introduction to the Laplace transform and even a lesson on how to calculate Laplace expressions by a simple method of comparison.

polynomial third order complex a method for solving s system of linear equations which uses. Solve differential equation using Laplace transform: Step by step laplace transform calculator easy algebra. Afterwards we will explain the calculations in a list of steps and we will finish by solving a few examples on the topic. In order to calculate such result, we will first compute the two main equations that will be used throughout the process, these equations which we recommend to learn and keep them at hand, are the ones shown in equation 6. This is exactly the case for our lesson of today, where we will use the Laplace transformation in order to decompose a higher order linear differential equation, separate its terms, simplify, and then work them through to obtain an expression for the implicit solution of the differential equation. It has been mentioned before on the introductory lesson, that mathematically speaking, we use the term transformations when referring to clever tricks in math that will allows to change a problem from higher level methodology into something simpler, such as algebra. Laplace transform for a function f(t) where t>=0.


 0 kommentar(er)
0 kommentar(er)
